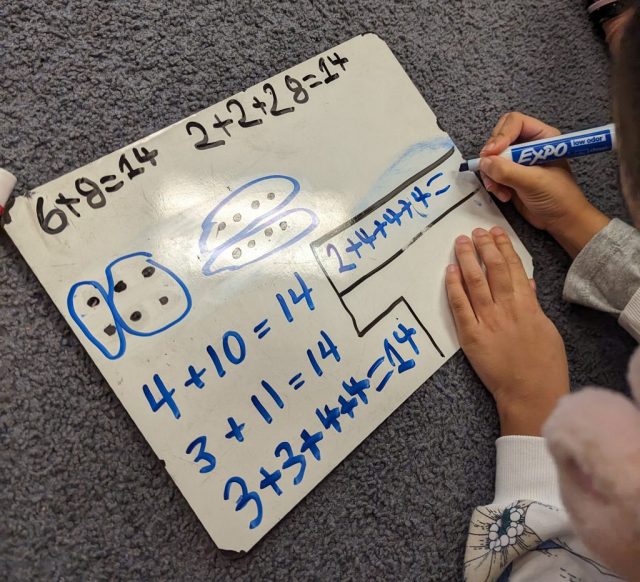Learning adventures in a Grade 2 French Immersion classroom
We wrap up our measurement unit this month. This week, we practiced measuring length (la longueur) with non-standard units of our own choosing.
Students learned that they need to use multiple copies of the units for the measurement to be accurate. For example, if choosing markers as a non-standard measurement unit, they needed to use the same markers.
Students were asked to first estimate the length of a given object and then measure it out. It’s been a fun math activity!
In Term 2, we continue to develop our number sense with a strong focus on addition up to 100.
What is number sense? You can think of it as “a person’s ability to understand, relate, and connect numbers.”
Our math learning takes form in different ways – worksheets, open-ended morning questions, picture books, games, drills (Chrono Maths), and number routines.
One such number routine is the ‘problem of the day’. This week, students have been reflecting on their addition strategies.
We’ve been using Carole Fullerton’s Good Questions: A Year of Open-Ended Math Problems for Grades 2-4 for daily discussion.
Open-ended questions are low-risk and inclusive to all learners. They invite multiple solutions and rich discussion. The class understands that so long as the answer ‘makes sense’ (ie. is logical), it is up for group consideration.
Students practice both creative and critical thinking skills during this activity, helping make math more dynamic. They also develop their communication skills as they might be asked to share their math thinking in different ways – symbolically, concretely, and/or verbally.
Open-ended questions include more students in the act of problem-solving. The more often we expose students to these key math ideas the more fluent they become; the more we recognize and celebrate different problem-solving approaches, the smarter the collective becomes. (Fullerton)
As the school year progresses, we’ll continue to build on this and other number routines.
We started playing a new game in PE this week. Students aim to win the biggest number of points by throwing beanbags into the hoop.
Yellow beanbags are worth 1 point each. Red beanbags are worth 10 points each.
This simple game has students practice a number of skills.
Students practice spatial reasoning in assessing how hard or soft to throw a bean bag for it to land on target.
They practice personal and social responsibility in participating in the game and encouraging their classmates.
Finally, they practice creative and critical thinking in counting beanbags to figure out final scores.
We’ve started exploring base 10 materials this week. We’re exploring this Big Idea from the Grade 2 curriculum: “Numbers to 100 represent quantities that can be decomposed into 10s and 1s.”
We’re learning this content regarding place value:
understanding of 10s and 1s
understanding the relationship between digit places and their value, to 99 (e.g., the digit 4 in 49 has the value of 40)
decomposing two-digit numbers into 10s and 1s
As seen in the photo above, students had fun exploring even bigger numbers. They noted how the number 10 is important in each unit, ex. 10 ones make one ten, 10 tens make one hundred, etc.
We’ve been learning relevant math vocabulary such as une unité (a one), une dizaine (a ten), une centaine (a hundred), or valeur de position (place value).
Students are learning to choose appropriate books for themselves. Every week, they’re encouraged to switch them to be more exposed to new stories.
We read daily in different ways – lecture avec un.e ami.e (read with a friend), lecture (teacher readaloud), lecture à soi (read to self), and lecture en groupe (group readaloud), so regularly changing one’s books is essential to sustain interest.
When we read to ourselves, we have a special routine in deciding how long we’ll try to read. We use ‘real world’ math in deciding just how many minutes we want to read on a given day. We use number lines to track different lengths of time in minutes.
Once decided, we aim to read that number of minutes following the three basic rules below. After the session, we use a chart to record how many minutes we’ve successfully read.
We know we’ve been successful if we’ve stayed in one spot, remained quiet, and read. Simple yet so hard sometimes!
This week, we’ve been focusing on estimation. Students are learning to estimate reasonably by looking for groups of 2, 5, or 10 and then using that quantity to estimate the total quantity.
Students’ impulse is to count immediately, but they’re learning that estimating is an important math skill in itself.
Today, they did an estimation activity with a partner. In each Ziploc bag, there were up to 50 objects. They were asked to look for a referent, estimate, and then count with ten frames.
Interestingly, many pairs estimated the exact number of objects in their Ziploc. 🤔 We’ll continue to practice estimating to fully understand what it means.
Consider creating your own ‘counting collection’ at home. It’s a great way to practice skip counting too!
We found a way to talk about ‘real world’ math today when talking about Halloween candy. Students shared what rules they have at home. How many candies are they allowed to eat per day? It then led to an important discussion about equality and value. What does the ‘equal sign’ represent in an equation?
Sometimes, we get inspired by nature to make art. In this math activity, students were asked to create 2D picture art with sticks. They were asked to only use 12 sticks and for them to be as straight as possible. Then, they were asked to identify shapes within their art.
We counted sides and noted the number of vertices. We practiced using words like un triangle, un carré, un rectangle…We started identifying special polygon shapes too, like un hexagone.
We ran out of time, but it would have been interesting to extend this activity by only using ‘small’ sticks. How would their picture art change? What if a range of stick lengths were used instead? Would that change the picture again?
This activity was taken from the fantastic book, Messy Maths, by Juliet Robertson.
Math routines are an important part of our math program. One such routine is called “Which One Doesn’t Belong?” or WODB in English. In class, we’ve translated it to Quel est l’intrus?
Students must identify and explain which image doesn’t belong. There may be a few possible answers. Rather than it be about finding the ‘right’ answer, the focus is on explaining why their answer makes sense to them.
In math, we’re learning that we need to be precise and logical with our ideas. Students practice a few core competencies in this activity – communication, critical thinking, and creative thinking.
Recently, on a hike, I spotted four different mushrooms. Together, we discussed which one didn’t belong.
One student reasoned that Mushroom #1 didn’t belong because it was more narrow than the others. Another said that Mushroom #4 didn’t belong because it was the only one growing on the ground rather than on trees. Someone else added that Mushroom #4 was the only one that grew alone rather than in clusters.
This math routine had us talk about form, size, colour, quantity, and orientation. Not only were we thinking like mathematicians but also like scientists!
We were curious to identify these local fungi. With the help of books and the Internet, we think:
Finally, we talked safety. We understood to never touch or eat wild mushrooms. There are too many lookalike mushrooms, and it’s often tricky to tell apart poisonous ones from non-poisonous ones.
Students understood that it’s always best to simply observe a mushroom. In doing so, we respect their place in our local ecosystems and leave them be.
